25 The Nature of Light
LumenLearning
Properties of Waves and Light
In many cases, the properties of light can be explained as a wave, as was shown in Young’s double-slit experiment.
LEARNING OBJECTIVES
Discuss how wave motion arises and its measurable properties, noting the conclusions of Young’s double slit experiment
KEY TAKEAWAYS
Key Points
- Wave motion arises when a periodic disturbance of some kind is propagated through an elastic medium. Pressure variations through air, transverse motions along a guitar string, or variations in the intensities of the local electric and magnetic fields in space, known as electromagnetic radiation, are all examples of waves.
- There are three measurable properties of wave motion: amplitude, wavelength, and frequency.
- A definitive experiment was Young’s double slit experiment, which demonstrated that light shined at two slits in a screen show an interference pattern characteristic of waves of light, rather than particles.
- The phase associated with a wave is also important in describing certain phenomena.
- The velocity of a wave is the product of the wavelength and the frequency.
Key Terms
- amplitude: The maximum value of the variable reached in either direction.
- wave: A shape that alternatively varies between a maximum in two opposite directions.
- frequency: The number of vibrations per second.
- wavelength: The distance traveled by the wave in a full period (1/frequency).
In this section, we will focus on the wave-like properties of light. While you will later learn about wave/particle duality (how light behaves as both a wave and a particle at the same time), here we shall discuss the wave nature of light and the experimental effects of this behavior.
Introduction to Wave Motion
Wave motion arises when a periodic disturbance of some kind is propagated through a medium. Pressure variations through air, transverse motions along a guitar string, or variations in the intensities of the local electric and magnetic fields in space, which constitute electromagnetic radiation, are all typical examples of wave motion. For each medium, there is a characteristic velocity at which the disturbance travels.
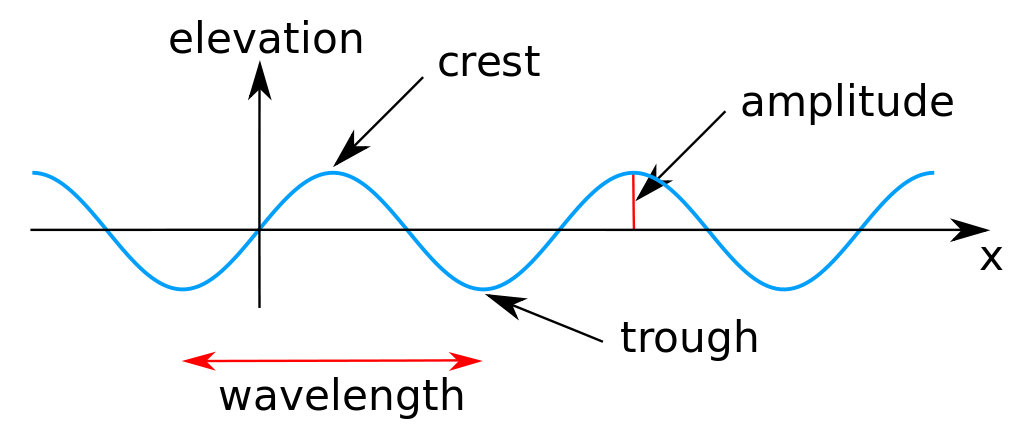
There are three measurable properties of wave motion: amplitude, wavelength, and frequency (the number of vibrations per second). The relation between the wavelength λ (Greek lambda) and frequency of a wave ν (Greek nu) is determined by the propagation velocity v, such that
[latex]v = \nu \lambda[/latex]
For light, this equation becomes
[latex]\nu = \frac{c}{\lambda}[/latex]
where c is the speed of light, 2.998 x 108 m/s.
When utilizing these equations to determine wavelength, frequency, or velocity by manipulation of the equation, it is important to note that wavelengths are expressed in units of length, such as meters, centimeters, nanometers, etc; and frequency is typically expressed as megahertz or hertz (s–1).
EXAMPLE
What is the wavelength of the musical note A = 440 hz when it is propagated through air in which the velocity of sound is 343 m s–1?
λ = v (343 m s-1)/ v(440 s–1) = 0.780 m
Young’s Double-Slit Experiment
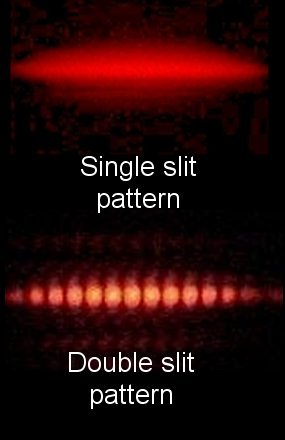
In the early 19th century, English scientist Thomas Young carried out the famous double-slit experiment (also known as Young’s experiment), which demonstrated that a beam of light, when split into two beams and then recombined, will show interference effects that can only be explained by assuming that light is a wavelike disturbance. If light consisted strictly of ordinary or classical particles, and these particles were fired in a straight line through a slit and allowed to strike a screen on the other side, we would expect to see a pattern corresponding to the size and shape of the slit. However, when this single-slit experiment is actually performed, the pattern on the screen is a diffraction pattern in which the light is spread out. The smaller the slit, the greater the angle of spread.
In the early 19th century, English scientist Thomas Young carried out the famous double-slit experiment (also known as Young’s experiment), which demonstrated that a beam of light, when split into two beams and then recombined, will show interference effects that can only be explained by assuming that light is a wavelike disturbance. If light consisted strictly of ordinary or classical particles, and these particles were fired in a straight line through a slit and allowed to strike a screen on the other side, we would expect to see a pattern corresponding to the size and shape of the slit. However, when this single-slit experiment is actually performed, the pattern on the screen is a diffraction pattern in which the light is spread out. The smaller the slit, the greater the angle of spread.
Similarly, if light consisted strictly of classical particles and we illuminated two parallel slits, the expected pattern on the screen would simply be the sum of the two single-slit patterns. In actuality, however, the pattern changes to one with a series of alternating light and dark bands.
When Thomas Young first demonstrated this phenomenon, it indicated that light consists of waves, as the distribution of brightness can be explained by the alternately additive and subtractive interference of wavefronts. Young’s experiment, performed in the early 1800’s, played a vital part in the acceptance of the wave theory of light, superseding the corpuscular theory of light proposed by Isaac Newton, which had been the accepted model of light propagation in the 17th and 18th centuries. Almost a century later, in 1905, Albert Einstein’s Nobel-Prize winning research into the photoelectric effect demonstrated that light can behave as if it is composed of discrete particles under certain conditions. These seemingly contradictory discoveries made it necessary to go beyond classical physics and take the quantum nature of light into account.
Electromagnetic Spectrum
The electromagnetic spectrum is the range of all possible frequencies of electromagnetic radiation.
LEARNING OBJECTIVES
Calculate frequency or photon energy, identify the three physical properties of electromagnetic waves
KEY TAKEAWAYS
Key Points
- The electromagnetic spectrum includes common regimes such as ultraviolet, visible, microwave, and radio waves.
- Electromagnetic waves are typically described by any of the following three physical properties: frequency (ν), wavelength (λ), or intensity (I). Light quanta are typically described by frequency (ν), wavelength (λ), or photon energy (E). The spectrum can be ordered according to frequency or wavelength.
- Electromagnetic radiation interacts with matter in different ways in different parts of the spectrum. The types of interaction can range from electronic excitation to molecular vibration depending on the different types of radiation, such as ultraviolet, X-rays, microwaves, and infrared radiation.
Key Terms
- gamma ray: Electromagnetic radiation of high frequency and therefore high energy per photon.
- spectrum: A range of colors representing light (electromagnetic radiation) of contiguous frequencies; hence electromagnetic spectrum, visible spectrum, ultraviolet spectrum, etc.
- photon: The quantum of light and other electromagnetic energy, regarded as a discrete particle having zero rest mass, no electric charge, and an indefinitely long lifetime.
Range of the Electromagnetic Spectrum
The electromagnetic spectrum is the range of all possible frequencies of electromagnetic radiation. The electromagnetic spectrum of an object has a different meaning: it is the characteristic distribution of electromagnetic radiation emitted or absorbed by that particular object.
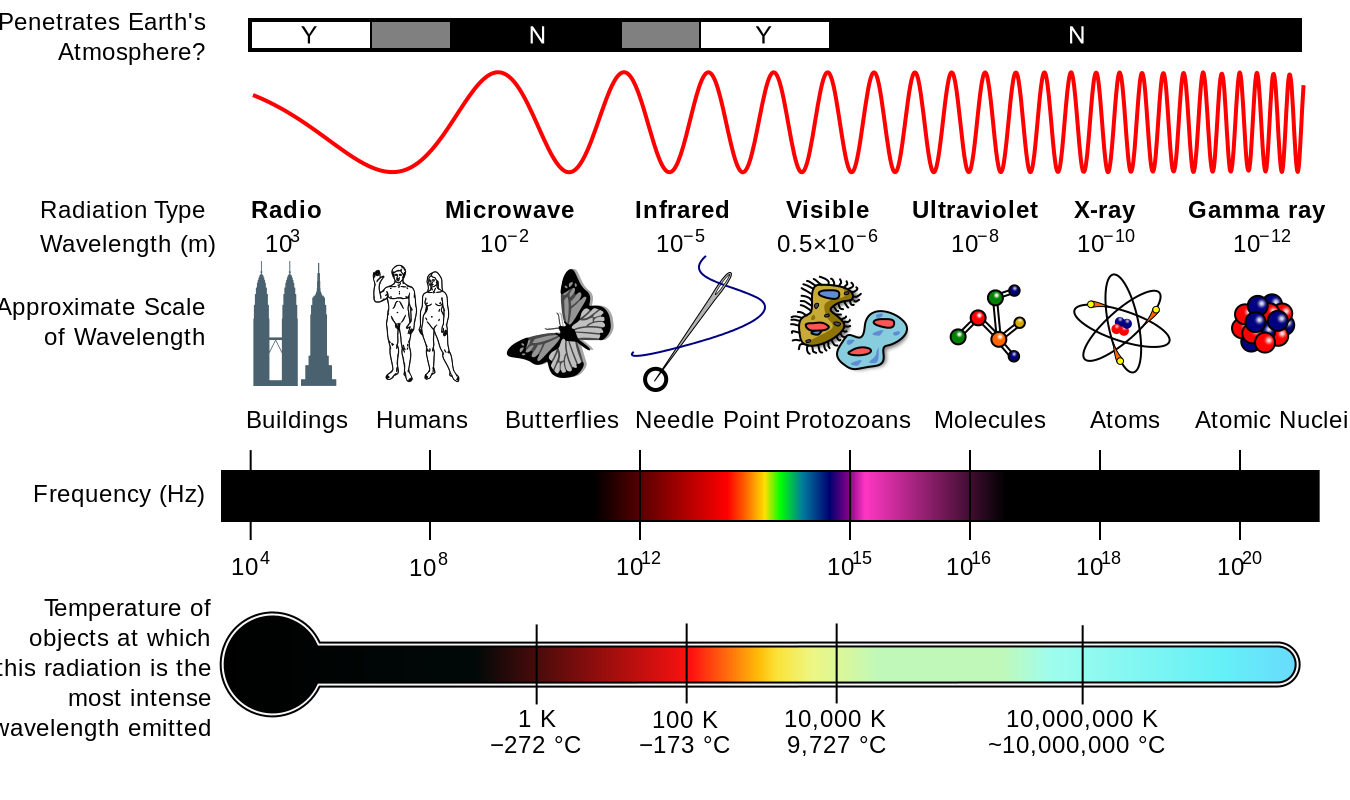
The electromagnetic spectrum extends from below the low frequencies used for modern radio communication to gamma radiation at the short-wavelength (high-frequency) end, covering wavelengths from thousands of kilometers down to a fraction of the size of an atom. The limit for long wavelengths is the size of the universe itself, while it is thought that the short wavelength limit is in the vicinity of the Planck length (1.616 x 10-35 m), although in principle the spectrum is infinite and continuous.
Most parts of the electromagnetic spectrum are used in science for spectroscopic and other probing interactions, as ways to study and characterize matter. In general, if the wavelength of electromagnetic radiation is of a similar size to that of a particular object (atom, electron, etc.), then it is possible to probe that object with that frequency of light. In addition, radiation from various parts of the spectrum has been found to have many other uses in communications and manufacturing.
Energy of Photon
Electromagnetic waves are typically described by any of the following three physical properties: the frequency (ν), the wavelength (λ), or photon energy (E). Frequencies observed in astronomy range from 2.4×1023Hz (1 GeV gamma rays ) down to the local plasma frequency of the ionized interstellar medium (~1 kHz). Wavelength is inversely proportional to wave frequency; hence, gamma rays have very short wavelengths that are a fraction of the size of atoms, whereas other wavelengths can be as long as the universe. Photon energy is directly proportional to the wave frequency, so gamma ray photons have the highest energy (around a billion electron volts), while radio wave photons have very low energy (around a femto-electron volt). These relations are illustrated by the following equations:
[latex]f = \frac{c}{\lambda} \text{ or } f = \frac{E}{h} \text{ or } E = \frac{hc}{\lambda}[/latex]
c = 299,792,458 m/s is the speed of light in vacuum
h = 6.62606896(33)×10−34 J s = 4.13566733(10)×10−15 eV s = Planck’s constant.
Whenever electromagnetic waves exist in a medium with matter, their wavelength is decreased. Wavelengths of electromagnetic radiation, no matter what medium they are traveling through, are usually quoted in terms of the vacuum wavelength, although this is not always explicitly stated. Generally, electromagnetic radiation is classified by wavelength into radio wave, microwave, infrared, the visible region we perceive as light, ultraviolet, X-rays, and gamma rays. The behavior of electromagnetic radiation depends on its wavelength. When electromagnetic radiation interacts with single atoms and molecules, its behavior also depends on the amount of energy per quantum (photon) it carries.
A.2.1 Describe the electromagnetic spectrum IB Chemistry SL – YouTube: This time with equations! Wave number = 1/wavelength in cm; Speed of light = wavelength x frequency; Energy = Planck’s constant x frequency. Dr Atkinson soon moved on to the un-needed gamma rays and improved them to delta rays!
Interference and Diffraction
Interference and diffraction are terms that describe a wave interacting with something that changes its amplitude, such as another wave.
LEARNING OBJECTIVES
Recognize the difference between constructive and destructive interference, and between interference and diffraction
KEY TAKEAWAYS
Key Points
- In physics, interference is a phenomenon in which two waves superimpose to form a resultant wave of greater or lower amplitude.
- Constructive interference occurs when the phase difference between the waves is a multiple of 2π, whereas destructive interference occurs when the difference is π, 3π, 5π, etc.
- Diffraction refers to various phenomena that occur when a wave encounters an obstacle. In classical physics, the diffraction phenomenon is described as the apparent bending of waves around small obstacles and the spreading out of waves past small openings.
Key Terms
- interference: An effect caused by the superposition of two systems of waves, such as a distortion on a broadcast signal due to atmospheric or other effects. In physics, interference is a phenomenon in which two waves superimpose to form a resultant wave of greater or lower amplitude.
- diffraction: The breaking up of an electromagnetic wave as it passes a geometric structure (e.g., a slit), followed by reconstruction of the wave by interference.
- amplitude: The maximum absolute value of some quantity that varies, especially a wave.
In physics, interference is a phenomenon in which two waves superimpose to form a resultant wave of greater or lower amplitude. Interference usually refers to the interaction of waves that are correlated or coherent with each other, either because they come from the same source or because they have the same (or nearly the same) frequency. Interference effects can be observed with all types of waves, including light, radio, acoustic, and surface water waves. In chemistry, the applications of interference to light are the most relevant to the study of matter.
Mechanism of Interference
The principle of superposition of waves states that when two or more waves are incident on the same point, the total displacement at that point is equal to the vector sum of the displacements of the individual waves. If a crest of a wave meets a crest of another wave of the same frequency at the same point, then the magnitude of the displacement is the sum of the individual magnitudes; this is known as constructive interference. If a crest of one wave meets a trough of another wave, then the magnitude of the displacements is equal to the difference in the individual magnitudes; this is known as destructive interference.
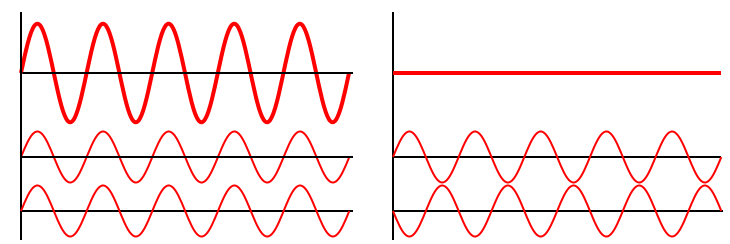
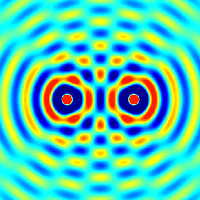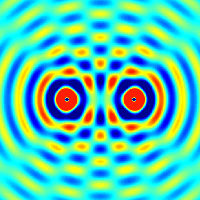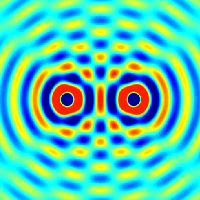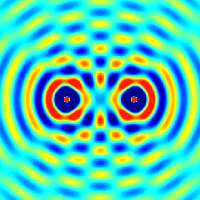
Constructive interference occurs when the phase difference between the waves is a multiple of 2π, whereas destructive interference occurs when the difference is π, 3π, 5π, etc.
If the difference between the phases is intermediate between these two extremes, then the magnitude of the displacement of the summed waves lies between the minimum and maximum values.
Consider, for example, what happens when two identical stones are dropped into a still pool of water at different locations. Each stone generates a circular wave propagating outwards from the point where the stone was dropped. When the two waves overlap, the net displacement at a particular point is the sum of the displacements of the individual waves. At some points, these will be in phase and will produce a maximum displacement. In other places, the waves will be in anti-phase and there will be no net displacement at these points. Thus, parts of the surface will be stationary.
Planck’s Quantum Theory
Max Planck suggested that the energy of light is proportional to its frequency, also showing that light exists in discrete quanta of energy.
LEARNING OBJECTIVES
Calculate the energy element E=hv, using Planck’s Quantum Theory
KEY TAKEAWAYS
Key Points
- Until the late 19th century, Newtonian physics dominated the scientific worldview. However, by the early 20th century, physicists discovered that the laws of classical mechanics do not apply at the atomic scale.
- The photoelectric effect could not be rationalized based on existing theories of light, as an increase in the intensity of light did not lead to the same outcome as an increase in the energy of the light.
- Planck postulated that the energy of light is proportional to the frequency, and the constant that relates them is known as Planck’s constant (h). His work led to Albert Einstein determining that light exists in discrete quanta of energy, or photons.
Key Terms
- photoelectric effect: The emission of electrons from the surface of a material following the absorption of electromagnetic radiation.
- electromagnetic radiation: Radiation (quantized as photons) consisting of oscillating electric and magnetic fields oriented perpendicularly to each other, moving through space.
In the late 18th century, great progress in physics had been made. Classical Newtonian physics at the time was widely accepted in the scientific community for its ability to accurately explain and predict many phenomena. However, by the early 20th century, physicists discovered that the laws of classical mechanics are not applicable at the atomic scale, and experiments such as the photoelectric effect completely contradicted the laws of classical physics. As a result of these observations, physicists articulated a set of theories now known as quantum mechanics. In some ways, quantum mechanics completely changed the way physicists viewed the universe, and it also marked the end of the idea of a clockwork universe (the idea that universe was predictable).
The Discovery of the Quantum
The wave model of electromagnetic radiation (EM) cannot account for something known as the photoelectric effect. This effect is observed when light focused on certain metals emits electrons. For each metal, there is a minimum threshold frequency of EM radiation at which the effect will occur. Replacement of light with twice the intensity and half the frequency will not produce the same outcome, contrary to what would be expected if light acted strictly as a wave. In that case, the effect of light would be cumulative—the light should add up, little by little, until it caused electrons to be emitted. Instead, there is a clear-cut minimum frequency of light that triggers electron ejection. The implication was that frequency is directly proportional to energy, with the higher light frequencies having more energy. This observation led to the discovery of the minimum amount of energy that could be gained or lost by an atom. Max Planck named this minimum amount the “quantum,” plural “quanta,” meaning “how much.” One photon of light carries exactly one quantum of energy.
Planck is considered the father of the Quantum Theory. According to Planck: [latex]E = h \nu[/latex], where h is Planck’s constant (6.62606957(29) x 10-34 J s), ν is the frequency, and E is energy of an electromagnetic wave. Planck (cautiously) insisted that this was simply an aspect of the processes of absorption and emission of radiation and had nothing to do with the physical reality of the radiation itself. However, in 1905, Albert Einstein reinterpreted Planck’s quantum hypothesis and used it to explain the photoelectric effect, in which shining light on certain materials can eject electrons from the material.
More Evidence for a Particle Theory of Energy
When an electric current is passed through a gas, some of the electrons in the gas molecules move from their ground energy state to an excited state that is further away from their nuclei. When the electrons return to the ground state, they emit energy of various wavelengths. A prism can be used to separate the wavelengths, making them easy to identify. If light acted only as a wave, then there should be a continuous rainbow created by the prism. Instead, there are discrete lines created by different wavelengths. This is because electrons release specific wavelengths of light when moving from an excited state to the ground state.

The Photoelectric Effect
The photoelectric effect is the propensity of high-energy electromagnetic radiation to eject electrons from a given material.
LEARNING OBJECTIVES
Explain the the photoelectric effect and understand its mathematical description
KEY TAKEAWAYS
Key Points
- In the photoelectric effect, electrons are emitted from matter (typically metals and non-metallic solids ) as a consequence of their absorption of energy from electromagnetic radiation of high frequency (short wavelength), such as ultraviolet light.
- When electromagnetic radiation interacts with an atom, it either excites electrons to a higher energy level known as an excited state, or, if the energy of the light is sufficiently high, it can ionize the atom by removing the electron.
- For a given metal, there exists a certain minimum frequency of incident radiation below which no photoelectrons are emitted. This frequency is called the threshold frequency.
Key Terms
- work function: The minimum energy needed to remove an electron from the surface of a material.
- stopping voltage: The voltage required to completely balance the kinetic energy of electrons ejected from a material’s surface.
In the photoelectric effect, electrons are emitted from matter (metals and non-metallic solids, liquids, or gases) as a consequence of their absorption of energy from electromagnetic radiation of high frequency (short wavelength), such as ultraviolet radiation. Electrons emitted in this manner may be referred to as photoelectrons. This phenomenon was first observed by Heinrich Hertz in 1887.

The photoelectric effect has been demonstrated using light with energies from a few electronvolts (eV) to over 1 MeV in high atomic number elements. Study of the photoelectric effect led to an improved understanding of quantum mechanics as well as an appreciation of the wave-particle duality of light. It also led to Max Planck’s discovery of quanta ([latex]E = h \nu[/latex]), which links frequency ([latex]\nu[/latex]) with photon energy (E).
Planck’s constant, h, is also known as “the quantum of action.” It is a subatomic-scale constant and is one of the smallest constants used in physics. Other phenomena where light affects the movement of electric charges include the photoconductive effect (also known as photoconductivity or photoresistivity), the photovoltaic effect, and the photoelectrochemical effect.
Emission Mechanism
All atoms have their electrons in orbitals with well-defined energy levels. When electromagnetic radiation interacts with an atom, it can excite the electron to a higher energy level, which can then fall back down, returning to the ground state. However, if the energy of the light is such that the electron is excited above energy levels associated with the atom, the electron can actually break free from the atom leading to ionization of the atom. This, in essence, is the photoelectric effect.
The photons of a beam of light have a characteristic energy proportional to the frequency of the light. In the photoemission process, if an electron within some material absorbs the energy of one photon and acquires more energy than the work function of the material (the electron binding energy), it is ejected. If the photon energy is too low, the electron is unable to escape the material. Increasing the intensity of the light increases the number of photons in the beam of light and thus increases the number of electrons excited but does not increase the energy that each electron possesses. The energy of the emitted electrons does not depend on the intensity of the incoming light (the number of photons), only on the energy or frequency of the individual photons. It is strictly an interaction between the incident photon and the outermost electron.
Electrons can absorb energy from photons when irradiated, but they usually follow an all-or-nothing principle. Typically, one photon is either energetic enough to cause emission of an electron or the energy is lost as the atom returns back to the ground state. If excess photon energy is absorbed, some of the energy liberates the electron from the atom and the rest contributes to the electron’s kinetic energy as a free particle.
Experimental Observations of Photoelectric Emission
For a given metal, there exists a certain minimum frequency of incident radiation below which no photoelectrons are emitted. This frequency is called the threshold frequency. Increasing the frequency of the incident beam and keeping the number of incident photons fixed (resulting in a proportionate increase in energy) increases the maximum kinetic energy of the photoelectrons emitted. The number of electrons emitted also changes because the probability that each impacting photon results in an emitted electron is a function of the photon energy. However, if just the intensity of the incident radiation is increased, there is no effect on the kinetic energies of the photoelectrons.
For a given metal and frequency of incident radiation, the rate at which photoelectrons are ejected is directly proportional to the intensity of the incident light. An increase in the intensity of the incident beam (keeping the frequency fixed) increases the magnitude of the photoelectric current, though the stopping voltage remains the same. The time lag between the incidence of radiation and the emission of a photoelectron is very small, less than 10−9 second, and is unaffected by intensity changes.
Photomultipliers
Photomultipliers are extremely light-sensitive vacuum tubes with a photocathode coated onto part (an end or side) of the inside of the envelope. The photocathode contains combinations of materials, such as caesium, rubidium, and antimony, specially selected to provide a low work function, so when illuminated by even very low levels of light, the photocathode readily releases electrons. By means of a series of electrodes (dynodes) at ever-higher potentials, these electrons are accelerated and substantially increased in number through secondary emission to provide a readily detectable output current. Photomultipliers are still commonly used wherever low levels of light must be detected.
LICENSES AND ATTRIBUTIONS
CC LICENSED CONTENT, SHARED PREVIOUSLY
- Curation and Revision. Provided by: Boundless.com. License: CC BY-SA: Attribution-ShareAlike
CC LICENSED CONTENT, SPECIFIC ATTRIBUTION
- Light, particles and waves. Provided by: Steve Lower’s Website. Located at: http://www.chem1.com/acad/webtext/atoms/atpt-2.html. License: CC BY: Attribution
- Double-slit experiment. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/Double-slit_experiment. License: CC BY-SA: Attribution-ShareAlike
- wave. Provided by: Wiktionary. Located at: http://en.wiktionary.org/wiki/wave. License: CC BY-SA: Attribution-ShareAlike
- Sine_wave_amplitude.svg. Provided by: Wikimedia. Located at: https://commons.wikimedia.org/wiki/File:Sine_wave_amplitude.svg. License: CC BY-SA: Attribution-ShareAlike
- Single and double slit 4. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/File:Single_and_double_slit_4.jpg. License: CC BY-SA: Attribution-ShareAlike
- Electromagnetic spectrum. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/Electromagnetic_spectrum. License: CC BY-SA: Attribution-ShareAlike
- spectrum. Provided by: Wiktionary. Located at: http://en.wiktionary.org/wiki/spectrum. License: CC BY-SA: Attribution-ShareAlike
- photon. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/photon. License: CC BY-SA: Attribution-ShareAlike
- gamma ray. Provided by: Wiktionary. Located at: http://en.wiktionary.org/wiki/gamma_ray. License: CC BY-SA: Attribution-ShareAlike
- Sine_wave_amplitude.svg. Provided by: Wikimedia. Located at: https://commons.wikimedia.org/wiki/File:Sine_wave_amplitude.svg. License: CC BY-SA: Attribution-ShareAlike
- Single and double slit 4. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/File:Single_and_double_slit_4.jpg. License: CC BY-SA: Attribution-ShareAlike
- A.2.1 Describe the electromagnetic spectrum IB Chemistry SL – YouTube. Located at: http://www.youtube.com/watch?v=ge6D7vT1fjo. License: Public Domain: No Known Copyright. License Terms: Standard YouTube license
- File:EM Spectrum Properties edit.svg – Wikipedia, the free encyclopedia. Provided by: Wikipedia. Located at: http://en.wikipedia.org/w/index.php?title=File:EM_Spectrum_Properties_edit.svg&page=1. License: CC BY-SA: Attribution-ShareAlike
- Interference (wave propagation). Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/Interference_(wave_propagation). License: CC BY-SA: Attribution-ShareAlike
- Diffraction. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/Diffraction. License: CC BY-SA: Attribution-ShareAlike
- interference. Provided by: Wiktionary. Located at: http://en.wiktionary.org/wiki/interference. License: CC BY-SA: Attribution-ShareAlike
- amplitude. Provided by: Wiktionary. Located at: http://en.wiktionary.org/wiki/amplitude. License: CC BY-SA: Attribution-ShareAlike
- diffraction. Provided by: Wiktionary. Located at: http://en.wiktionary.org/wiki/diffraction. License: CC BY-SA: Attribution-ShareAlike
- Sine_wave_amplitude.svg. Provided by: Wikimedia. Located at: https://commons.wikimedia.org/wiki/File:Sine_wave_amplitude.svg. License: CC BY-SA: Attribution-ShareAlike
- Single and double slit 4. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/File:Single_and_double_slit_4.jpg. License: CC BY-SA: Attribution-ShareAlike
- A.2.1 Describe the electromagnetic spectrum IB Chemistry SL – YouTube. Located at: http://www.youtube.com/watch?v=ge6D7vT1fjo. License: Public Domain: No Known Copyright. License Terms: Standard YouTube license
- File:EM Spectrum Properties edit.svg – Wikipedia, the free encyclopedia. Provided by: Wikipedia. Located at: http://en.wikipedia.org/w/index.php?title=File:EM_Spectrum_Properties_edit.svg&page=1. License: CC BY-SA: Attribution-ShareAlike
- Two sources interference. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/File:Two_sources_interference.gif. License: Public Domain: No Known Copyright
- File:Interference of two waves.svg – Wikipedia, the free encyclopedia. Provided by: Wikipedia. Located at: http://en.wikipedia.org/w/index.php?title=File:Interference_of_two_waves.svg&page=1. License: CC BY-SA: Attribution-ShareAlike
- electromagnetic radiation. Provided by: Wiktionary. Located at: http://en.wiktionary.org/wiki/electromagnetic_radiation. License: CC BY-SA: Attribution-ShareAlike
- General Chemistry/Introduction to Quantum Theory. Provided by: Wikibooks. Located at: http://en.wikibooks.org/wiki/General_Chemistry/Introduction_to_Quantum_Theory. License: CC BY-SA: Attribution-ShareAlike
- Quantum mechanics. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/Quantum_mechanics. License: CC BY-SA: Attribution-ShareAlike
- Boundless. Provided by: Boundless Learning. Located at: http://www.boundless.com//physics/definition/photoelectric-effect. License: CC BY-SA: Attribution-ShareAlike
- Plank Constant. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/Planck_constant. License: CC BY-SA: Attribution-ShareAlike
- Sine_wave_amplitude.svg. Provided by: Wikimedia. Located at: https://commons.wikimedia.org/wiki/File:Sine_wave_amplitude.svg. License: CC BY-SA: Attribution-ShareAlike
- Single and double slit 4. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/File:Single_and_double_slit_4.jpg. License: CC BY-SA: Attribution-ShareAlike
- A.2.1 Describe the electromagnetic spectrum IB Chemistry SL – YouTube. Located at: http://www.youtube.com/watch?v=ge6D7vT1fjo. License: Public Domain: No Known Copyright. License Terms: Standard YouTube license
- File:EM Spectrum Properties edit.svg – Wikipedia, the free encyclopedia. Provided by: Wikipedia. Located at: http://en.wikipedia.org/w/index.php?title=File:EM_Spectrum_Properties_edit.svg&page=1. License: CC BY-SA: Attribution-ShareAlike
- Two sources interference. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/File:Two_sources_interference.gif. License: Public Domain: No Known Copyright
- File:Interference of two waves.svg – Wikipedia, the free encyclopedia. Provided by: Wikipedia. Located at: http://en.wikipedia.org/w/index.php?title=File:Interference_of_two_waves.svg&page=1. License: CC BY-SA: Attribution-ShareAlike
- Nitrogen.Spectrum.Vis. Provided by: Wikimedia. Located at: http://commons.wikimedia.org/wiki/File:Nitrogen.Spectrum.Vis.jpg. License: Public Domain: No Known Copyright
- Wavelength. Provided by: Wikimedia. Located at: http://commons.wikimedia.org/wiki/File:Wavelength.png. License: CC BY-SA: Attribution-ShareAlike
- Photoelectric effect. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/Photoelectric_effect. License: CC BY-SA: Attribution-ShareAlike
- work function. Provided by: Wiktionary. Located at: http://en.wiktionary.org/wiki/work_function. License: CC BY-SA: Attribution-ShareAlike
- Sine_wave_amplitude.svg. Provided by: Wikimedia. Located at: https://commons.wikimedia.org/wiki/File:Sine_wave_amplitude.svg. License: CC BY-SA: Attribution-ShareAlike
- Single and double slit 4. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/File:Single_and_double_slit_4.jpg. License: CC BY-SA: Attribution-ShareAlike
- A.2.1 Describe the electromagnetic spectrum IB Chemistry SL – YouTube. Located at: http://www.youtube.com/watch?v=ge6D7vT1fjo. License: Public Domain: No Known Copyright. License Terms: Standard YouTube license
- File:EM Spectrum Properties edit.svg – Wikipedia, the free encyclopedia. Provided by: Wikipedia. Located at: http://en.wikipedia.org/w/index.php?title=File:EM_Spectrum_Properties_edit.svg&page=1. License: CC BY-SA: Attribution-ShareAlike
- Two sources interference. Provided by: Wikipedia. Located at: http://en.wikipedia.org/wiki/File:Two_sources_interference.gif. License: Public Domain: No Known Copyright
- File:Interference of two waves.svg – Wikipedia, the free encyclopedia. Provided by: Wikipedia. Located at: http://en.wikipedia.org/w/index.php?title=File:Interference_of_two_waves.svg&page=1. License: CC BY-SA: Attribution-ShareAlike
- Nitrogen.Spectrum.Vis. Provided by: Wikimedia. Located at: http://commons.wikimedia.org/wiki/File:Nitrogen.Spectrum.Vis.jpg. License: Public Domain: No Known Copyright
- Wavelength. Provided by: Wikimedia. Located at: http://commons.wikimedia.org/wiki/File:Wavelength.png. License: CC BY-SA: Attribution-ShareAlike
- File:Photoelectric effect.svg – Wikipedia, the free encyclopedia. Provided by: Wikipedia. Located at: http://en.wikipedia.org/w/index.php?title=File:Photoelectric_effect.svg&page=1. License: CC BY-SA: Attribution-ShareAlike
This chapter is an adaptation of the chapter “The Nature of Light” in Boundless Chemistry by LumenLearning and is licensed under a CC BY-SA 4.0 license.
The maximum value of the variable reached in either direction.
A shape that alternatively varies between a maximum in two opposite directions.
The number of vibrations per second.
The distance traveled by the wave in a full period (1/frequency).
Electromagnetic radiation of high frequency and therefore high energy per photon.
A range of colors representing light (electromagnetic radiation) of contiguous frequencies; hence electromagnetic spectrum, visible spectrum, ultraviolet spectrum, etc.
The quantum of light and other electromagnetic energy, regarded as a discrete particle having zero rest mass, no electric charge, and an indefinitely long lifetime.
An effect caused by the superposition of two systems of waves, such as a distortion on a broadcast signal due to atmospheric or other effects. In physics, interference is a phenomenon in which two waves superimpose to form a resultant wave of greater or lower amplitude.
The breaking up of an electromagnetic wave as it passes a geometric structure (e.g., a slit), followed by reconstruction of the wave by interference.
The emission of electrons from the surface of a material following the absorption of electromagnetic radiation.
Radiation (quantized as photons) consisting of oscillating electric and magnetic fields oriented perpendicularly to each other, moving through space.
The minimum energy needed to remove an electron from the surface of a material.
The voltage required to completely balance the kinetic energy of electrons ejected from a material’s surface.

