15 Gas Laws

Boyle’s Law: Volume and Pressure

Boyle’s law describes the inverse relationship between the pressure and volume of a fixed amount of gas at a constant temperature.

Boyle’s Law

Boyle’s law (sometimes referred to as the Boyle-Mariotte law) states that the absolute pressure and volume of a given mass of confined gas are inversely proportional, provided the temperature remains unchanged within a closed system. This can be stated mathematically as follows:

[latex]P_1V_1 = P_2V_2[/latex]

History and Derivation of Boyle’s Law

The law was named after chemist and physicist Robert Boyle, who published the original law in 1662. Boyle showed that the volume of air trapped by a liquid in the closed short limb of a J-shaped tube decreased in exact proportion to the pressure produced by the liquid in the long part of the tube.

The trapped air acted much like a spring, exerting a force opposing its compression. Boyle called this effect “the spring of the air” and published his results in a pamphlet with that title. The difference between the heights of the two mercury columns gives the pressure (76 cm = 1 atm), and the volume of the air is calculated from the length of the air column and the tubing diameter.

The law itself can be stated as follows: for a fixed amount of an ideal gas kept at a fixed temperature, P (pressure) and V (volume) are inversely proportional—that is, when one doubles, the other is reduced by half.

Remember that these relations hold true only if the number of molecules (n) and the temperature (T) are both constant.

“Boyle’s Law”: An introduction to the relationship between pressure and volume and an explanation of how to solve gas problems with Boyle’s law.

Charles’s and Gay-Lussac’s Law: Temperature and Volume

Charles’s and Gay-Lussac’s law states that at constant pressure, temperature, and volume are directly proportional.

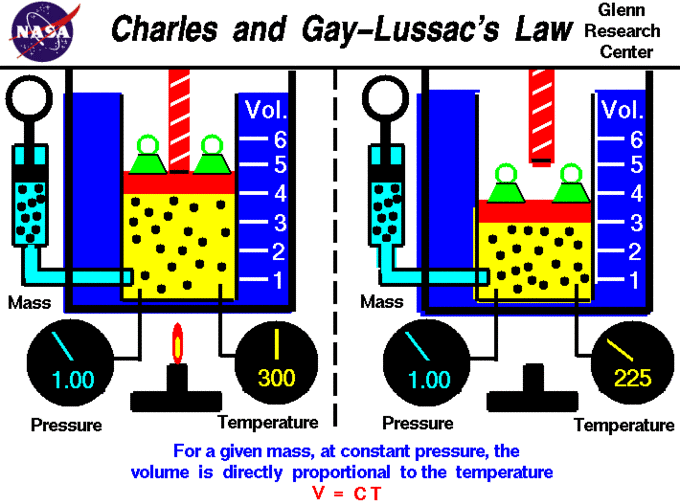
Charles’s and Guy-Lussac’s Law

Charles’s law describes the relationship between the volume and temperature of a gas. The law was first published by Joseph Louis Gay-Lussac in 1802, but he referenced unpublished work by Jacques Charles from around 1787. This law states that at constant pressure, the volume of a given mass of an ideal gas increases or decreases by the same factor as its temperature (in Kelvin); in other words, temperature and volume are directly proportional. Stated mathematically, this relationship is

[latex]\frac{V_1}{T_1} = \frac{V_2}{T_2}[/latex]

V vs. T Plot and Charles’s Law

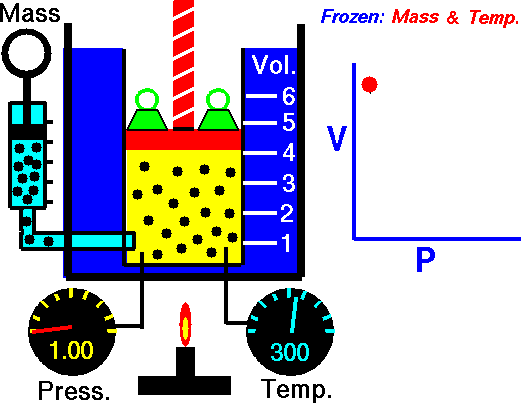
A visual expression of Charles’s and Gay-Lussac’s law is shown in a graph of the volume of one mole of an ideal gas as a function of its temperature at various constant pressures. The plots show that the ratio [latex]\frac{V}{T}[/latex] (and thus [latex]\frac{\Delta V}{\Delta T}[/latex]) is a constant at any given pressure. Therefore, the law can be expressed algebraically as [latex]\frac{\Delta V}{\Delta T} = \text{constant or } \frac{V_1}{T_1} = \frac{V_2}{T_2}[/latex].

Extrapolation to Zero Volume

If a gas contracts by 1/273 of its volume for each degree of cooling, it should contract to zero volume at a temperature of –273°C; this is the lowest possible temperature in the universe, known as absolute zero. This extrapolation of Charles’s law was the first evidence of the significance of this temperature.

Why Do the Plots for Different Pressures Have Different Slopes?

The lower a gas’s pressure, the greater its volume (Boyle’s law), so at low pressures, the fraction [latex]\frac{V}{273}[/latex] will have a larger value; therefore, the gas must “contract faster” to reach zero volume when its starting volume is larger.

“Charles’ Law”: Discusses the relationship between volume and temperature of a gas and explains how to solve problems using Charles’s law.

Avogadro’s Law: Volume and Amount

Avogadro’s law states that at the same temperature and pressure, equal volumes of different gases contain an equal number of particles.

Definition of Avogadro’s Law

Avogadro’s law (sometimes referred to as Avogadro’s hypothesis or Avogadro’s principle) is a gas law; it states that under the same pressure and temperature conditions, equal volumes of all gases contain the same number of molecules. The law is named after Amedeo Avogadro who, in 1811, hypothesized that two given samples of an ideal gas—of the same volume and at the same temperature and pressure—contain the same number of molecules; thus, the number of molecules or atoms in a specific volume of ideal gas is independent of their size or the molar mass of the gas. For example, 1.00 L of N₂ gas and 1.00 L of Cl₂ gas contain the same number of molecules at Standard Temperature and Pressure (STP).

Avogadro’s law is stated mathematically as:

[latex]\frac{V}{n} = k[/latex]

V is the volume of the gas, n is the number of moles of the gas, and k is a proportionality constant.

As an example, equal volumes of molecular hydrogen and nitrogen contain the same number of molecules and observe ideal gas behavior when they are at the same temperature and pressure. In practice, real gases show small deviations from the ideal behavior and do not adhere to the law perfectly; the law is still a useful approximation for scientists, however.

Significance of Avogadro’s Law

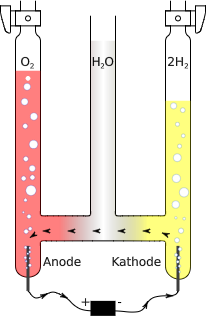
Discovering that the volume of a gas was directly proportional to the number of particles it contained was crucial in establishing the formulas for simple molecules at a time (around 1811) when the distinction between atoms and molecules was not clearly understood. In particular, the existence of diatomic molecules of elements such as H₂, O₂, and Cl₂ was not recognized until the results of experiments involving gas volumes were interpreted.

Early chemists calculated the molecular weight of oxygen using the incorrect formula HO for water. This led to the molecular weight of oxygen being miscalculated as 8, rather than 16. However, when chemists found that an assumed reaction of [latex]\text{H + Cl} \rightarrow \text{HCl}[/latex] yielded twice the volume of HCl, they realized hydrogen and chlorine were diatomic molecules. The chemists revised their reaction equation to be [latex]\text{H}_\text{2} \text{ + Cl}_\text{2} \rightarrow \text{2HCl}[/latex].

When chemists revisited their water experiment and their hypothesis that [latex]\text{HO } \rightarrow \text{ H + O}[/latex], they discovered that the volume of hydrogen gas consumed was twice that of oxygen. By Avogadro’s law, this meant that hydrogen and oxygen were combining in a 2:1 ratio. This discovery led to the correct molecular formula for water (H₂O) and the correct reaction [latex]\text{2H}_\text{2} \text{O} \rightarrow \text{2H}_\text{2} \text{ + O}_\text{2}[/latex].

“Avogadro’s Law”: Practice problems and examples looking at the relationship between the volume and amount of gas (number of moles) in a gas sample.