CHAPTER 2: MEASUREMENT

2-4: International System of Measurement: Length and Area

A Quick History of the International System (SI) of Measurement

The French Revolution began in May 1789 and was a period of radical political and societal change in France. The political climate and technological advances demanded reform of the units used for measurement. A decimalized measurement system was proposed that was based upon a length equal to one ten-millionth of the length of a quadrant of the earth’s meridian (i.e., one ten-millionth of the distance between the equator and the North Pole). This invariable standard for all the measures and all the weights [1] was adopted in 1790, creating a system that was, at once, simple and scientific. The unit of length was to be a portion of the Earth’s circumference. Measures for volume, capacity and mass were to be derived from the unit of length, thus relating the basic units of the system to each other and to nature. Furthermore, larger and smaller multiples of each unit were to be created by multiplying or dividing the basic units by 10 and its powers. This feature provided a great convenience to users of the system, by eliminating the need for such calculations as dividing by 16 (to convert ounces to pounds) or by 12 (to convert inches to feet). Similar calculations in the International System could be performed simply by shifting the decimal point. Thus, the International System is a decimal (base 10) system.

An Introduction to the International System (SI) of Measurement 

Since most of the world (including our scientific community in the US) uses the SI (from the French Le Système International d’Unités) or metric system, it is important to know how to measure using this system and how to identify appropriate units to use.

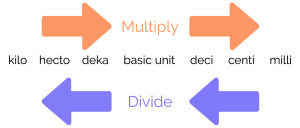
The SI uses prefixes along with a basic unit to measure length, capacity, and weight. The basic unit of length is the meter, capacity uses the liter, and mass uses the gram. Attaching a prefix to these basic units changes the measurement by a power of 10, so every measurement is related to the basic unit. The huge benefit of this is that converting from one unit to another is simply a case of multiplying or dividing by a power of 10.

The SI is a base-10 decimal number system. Each prefix changes the basic unit to be a power of 10 larger or smaller. Table 1 shows the metric prefixes attached to a basic unit. Moving left from the basic unit increases the basic unit by a power of 10, while moving right from the basic unit decreases the basic unit by a power of 10. 1 unit is 10 deci-units. 1 deci-unit is 10 centi-units. Therefore, 1 unit is 100 centi-units. On the other hand, 1 deci-unit is 0.1 unit  and 1 centi-unit is 0.01 unit.

Metric Prefixes kilounit hectounit dekaunit unit deciunit centiunit milliunit
Abbreviation k h da   d c m
Relative Size to basic unit 1000 100 10 1 0.1 0.01 0.001

 

Explore Icon Measuring Length

Length is a one-dimensional measure of how long something is. A metric ruler is used to measure the length of an object in meters, centimeters, and millimeters. Each meter is divided into 100 centimeters, and each centimeter is divided into 10 millimeters.

Due to the size of objects, different sizes of length units are used for measuring relatively larger or smaller objects. However, each unit used refers back to the meter, using prefixes appropriate to the length being measured.

 

Explore 1 – Expressing a length measure

1. What is an appropriate measure for each object:

a) a soup spoon

b) the distance between two cities

c) the length of a dining room table

d) the length of a fingernail

Solutions

a) centimeters or millimeters.    b) kilometers.    c) centimeters or meters.    d) millimeters

2. Read the measurement of each object from the ruler.

Penny against a ruler that reads 1.5 tenths of a centimeter past 1 centimeter

Pencil lying against rule with tip at 0 cm and end at 6 units past 3 cm

 

Solution

The penny’s edge lies between 1 and 2 mm after the 1 cm mark. The penny is 1.15 cm long. The pencil ends at 6 mm past the 3 cm mark, so the pencil is 3.6 cm long.

3. How many centimeters long is your right foot? Use a metric ruler to measure the length of your right foot. Express your answer in decimals to the tenths place.

 

 

Explore 2 – Basic length unit conversion

Perform the following unit conversions. Questions 1-3 show conversions from a larger unit to a smaller unit. Questions 4-6 show conversions from a smaller unit to a larger unit.

  1. Jenny’s height is 1.83 meters. Convert 1.83 meters to centimeters.

Solution

The prefix centi lies two prefixes to the right of the basic unit meter, so there are 100 cm in 1 m. Since we are converting from a larger to a smaller unit, there are more of the smaller unit so we multiply by 100 (equivalent to moving the decimal point 2 places to the right): 1.83m = 183cm.

  1. Orem City in Utah holds a 5km race. Convert 5 km to meters.

Solution

The prefix kilo lies three prefixes to the left of the basic unit meter, so there are 1000 m in 1 km. Since we are converting from a larger to a smaller unit, there are more of the smaller unit so we multiply by 1000 (equivalent to moving the decimal point 3 places to the right): 5 km = 5000 m.

  1. The diameter of a quarter coin is about 2.3 centimeters. Convert 2.3 centimeters to millimeters.

Solution

The prefix milli lies one prefix to the right of the prefix centi, so there are 10 mm in 1 cm. Since we are converting from a larger to a smaller unit, there are more of the smaller unit so we multiply by 10 (equivalent to moving the decimal point 1 place to the right): 2.3 cm = 23 mm.

  1. The diameter of a quarter coin is about 2.3 centimeters. Convert 2.3 centimeters to meters.

Solution

The prefix centi lies two prefixes to the right of the basic unit meter, so there are 100 cm in 1 m. Since we are converting from a smaller to a larger unit, there will be fewer of the larger unit so we divide by 100 (equivalent to moving the decimal point 2 places to the left): 2.3 cm = 0.023 m.

  1. The length of an ant is about 2mm. Convert 2mm to centimeters.

Solution

The prefix milli lies one prefix to the right of the prefix centi, so there are 10 mm in 1 cm. Since we are converting from a smaller to a larger unit, there will be fewer of the larger unit so to convert from mm to cm we divide by 10 (equivalent to moving the decimal point 1 place to the left): 2 mm = 0.2 cm.

  1. David Rudisha holds the world record for the men’s Olympic 800 meter race (1:40.91). Hicham El Guerrouj holds the world record for the men’s Olympic 1500 meter race (3:26.00). Daniel Komen holds the men’s world record for the Olympic 3000 meter race (7:20.67). Convert the race lengths from meters to kilometers.

Solution

To convert from the smaller unit meter to the larger unit kilometer, we divide by 1000: 800 m = 0.8 km; 1500 m = 1.5 km; 3000 m = 3 km.

 

Examples

  1. Paul has a height of 177.8 cm. What is his height in meters?
  2. A pencil case measures 21.4 cm by 8.5 cm by 4.3 cm. Write the dimensions in millimeters.
  3. The distance from Paris, France to London, England is 470.6 km. Write this distance in meters.

 

Show/Hide Answer
  1. We are converting from a smaller (cm) to a larger unit (m) so we divide: 177.8 cm = 1.778 meters
  2. We are converting from a larger (cm) to a smaller unit (mm) so we multiply: 21.4 cm = 214 mm; 8.5 cm = 85 mm; 4.3 cm = 43 mm
  3. We are converting from a larger (km) to a smaller unit (m) so we multiply: 470.6 km = 470,600 m.

 

 

Reflect Icon

 

  • How do you know whether you should multiply or divide by a power of 10 in metric conversion?
Show/Hide Answer

If we are converting from larger to smaller units, there are more of the smaller units so we multiply by a power of 10, where the power is equal to the number of prefixes to the right the smaller prefix is from the larger prefix. If we are converting from smaller to larger units, there are fewer of the larger units so we divide by a power of 10, where the power is equal to the number of prefixes to the left the larger prefix is from the smaller prefix.

Larger to smaller prefixes: Multiply Smaller to larger prefixes: divide

 

Practice Exercises – Length

  1. Piano key with ruler. Key measures from 0cm to 3.5 units past 2cmWhat is an appropriate measure for the length of a leaf on a plant?
  2. What is an appropriate measure for the height of a mountain above sea level? What is Mount Everest’s altitude in this unit?
  3. What is an appropriate measure for the length of a dollar bill? What is this length?
  4. What is the width of a white piano key?
  5. The distance from Toronto, ON to Vancouver, BC is 4,339,800 m. Write the distance in kilometers.
  6. Kara wears 6mm round garnet earrings. Convert this diameter to centimeters.
  7. Bailey wears a 2.5 cm wide collar. Write this measurement in millimeters.
  8. An Olympic-sized swimming pool has a lane length of 50 meters. Write this length in kilometers.

 

Show/Hide Answer
  1. Plants can be small or large so length should be measured in millimeters, centimeters or meters.
  2. Mountains are generally measured in meters. Mount Everest’s altitude is 8848.9 meters above sea level.
  3. A dollar bill can be measured in millimeters or centimeters. A dollar bill measures 156 mm = 15.6 cm.
  4. 2.35 cm
  5. We are converting from a smaller (m) to a larger unit (km) so we divide: 4,339,800 m = 4339.8 km.
  6. We are converting from a smaller (mm) to a larger unit (cm) so we divide: 6 mm = 0.6 cm
  7. We are converting from a larger (cm) to a smaller unit (km) so we multiply: 2.5 cm = 25 mm
  8. We are converting from a smaller (m) to a larger unit (km) so we divide: 50 m = 0.05 km

 

 

Explore Icon Measuring Area

Area is a measure of the amount of space inside a two-dimensional region. For example, a square that has the dimensions 1 centimeter by 1 centimeter has an area of 1 square centimeter or 1 cm2. A square that has the dimensions 1 meter by 1 meter has an area of 1 square meter or 1 m2. A square that has the dimensions 1 kilometer by 1 kilometer has an area of 1 square kilometer or 1 km2.

 

Explore 3 – Expressing an area measure

The area of a rectangle can be calculated using the formula Area=length \times width. The formula shows the number of squares in a row times the number of squares in a column. The result is the number of squares in a two-dimensional region. For example, a region with length 10 cm and width 5cm has an area of 10 · 5 = 50 squares where each square has the dimensions 1cm by 1cm. Since there are 50 1cm by 1cm squares, we say the area is 50 square centimeters or 50 cm2.

What is an appropriate measure for the area:

  1. A TV screen

Solution

TV dimensions are typically in tens or hundreds of centimeters. So area is measured in square centimeters or square meters.

  1. A pillow cover

Solution

Pillow cover dimensions are typically in centimeters. So area is in square centimeters.

  1. A parking lot

Solution

Parking lots are typically measured in meters, so area is in square meters.

  1. The area occupied by a city

Solution

City dimensions are typically measured in kilometers, so area is square kilometers.

Determine the area:

  1. An interior design studio suggests that 60 cm by 120 cm size floor tiles give a sense of wide-open space while the 30 cm by 120 cm size floor tiles are ideal for wood effect ceramic. The lobby of a ski resort in Park City (Utah) measures 10 m by 15 m. How many tiles are needed to cover the lobby for each of the two tile styles.

Solution

The lobby measures 10 m = 1000 cm by 15 m = 1500 cm, so Area = 1000 cm · 1500 cm = 1,500,000 cm2. The area of a tile of size 60 cm by 120 cm is 60cm · 120 cm = 7200 cm2. To find the number of tiles required we divide the total area of the lobby by the area covered by one tile: 1,500,000 cm2 ÷ 7200 cm2 = 208.33. Therefore, It takes 209 tiles with dimensions 60 cm by 120 cm to cover the lobby. The area of a tile with dimensions 30 cm by 120 cm is 30 cm · 120 cm = 3600 cm2. Therefore, the number of tiles needed = 1,500,000 cm2 ÷ 3600 cm2 = 416.67. Therefore, It takes 417 tiles with dimensions 30 cm by 120 cm to cover the lobby. Answer: It requires 209 tiles to cover the lobby using the tile with dimensions 60 cm by 120 cm. It requires 417 tiles to cover the lobby using the tile with dimensions 30 cm by 120 cm.

  1. A standard soccer field has a length of 100 meters and width of 64 meters. Calculate the area of the soccer field.

Solution

Area = 100m · 64m = 6400 m2.

  1. In Japan, tatami mat size is used for floor measurement, and the value of a house is calculated in terms of the number of tatami mats. The dimensions of a tatami mat are about 1.76 m by 0.88 m. a) Calculate the area of a tatami mat.  b) A traditional Japanese tearoom is the size of 4.5 tatami mats. What is the size of the tearoom in square meters?

Solution

a) The area of a tatami mat is (1.76 m)(0.88 m) = 1.5488 m2.

b) To determine the size of the tearoom, we multiply the area of one tatami mat by 4.5:  1.5488 m2 · 4.5 = 6.9696 m2.  The tearoom is about 7 m2 in size.

 

Explore 4 – Basic area unit conversion

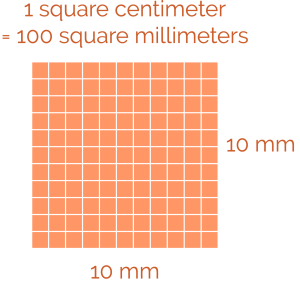
  1. Explain why 1 cm2 = 100 mm2. A picture may help you.10 by 10 squares
  2. Explain why 1 m2 = 10,000 cm2. A picture may help you.
  3. A piece of skin tissue is found at a crime scene. The size of the skin tissue is about 0.5 cm by 1.8 cm. Find the area of the tissue in square millimeters.
  4. The size of each tile in an outside courtyard has dimensions 120 cm by 120 cm. There are 160 such tiles in the courtyard. Determine the area of the courtyard in square meters.

Solutions

  1. There are ten 1 mm by 1 mm squares on each side of a square that is 1 cm by 1 cm (or 1 cm2). Therefore, there are 10 · 10 = 100 squares (i.e., 100 mm2) in 1 cm2.
  2. There are one hundred 1 cm by 1 cm squares on each side of a square that is 1m by 1 m (or 1 m2). Therefore, there are 100 · 100=10,000 cm2 in 1 m2.
  3. Since we want the answer to be in mm2, we can either work with the given measurements in cm to determine the area, then convert from cm2 to mm2, or we can convert cm to mm first, then determine the area in mm2. First way: Area 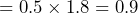=0.5\times1.8=0.9 cm2. To convert from cm2 to mm2, we multiply by 100 (1cm2 = 100mm2): 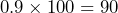0.9\times100=90 mm2. Second way: 0.5 cm = 5 mm and 1.8 cm = 18 mm. Therefore, the area 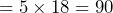=5\times18=90 mm2. Answer: 90 mm2.
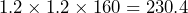  4. 120 cm = 1.2 m, so the area of each tile is 1.2\times1.2 m2. Consequently, the area of the courtyard with 160 tiles is 1.2\times1.2\times160=230.4 m2. Answer: 230.4 m2.

 

Reflect Icon

 

  • What is the meaning of “length” times “width” for finding the area of a two-dimensional region?
Show/Hide Answer

The meaning of length and width of a region is the number of squares on the longer side (length) and the shorter side (width) of a region. The meaning of “length” times “width” is the number of squares in a row times the number of squares in a column in order to find the total number of squares in the region.

  • Why are the units for area squared?
Show/Hide Answer

Area is a measure of the number of unit squares that make up a 2-dimensional space. The length and width have a basic unit of meter, so their product when multiplied has a basic unit of meter · meter = square meters.

 

Practice Exercises – Area

  1. What is an appropriate measure to calculate the area of a window?
  2. What is an appropriate measure to calculate the area of a rug?
  3. What is an appropriate measure to calculate the area of a book cover?
  4. The Mona Lisa, painted by Leonardo Da Vinci, measures 5.5 m by 3.9 m. Determine the area of this painting.
  5. A dollar bill measures 156 mm by 66.3 mm. Determine the area of a dollar bill in square millimeters and square centimeters.
  6. The face of the iPhone 14 measures 146.7 mm by 71.5 mm. Determine its area in square millimeters and square centimeters..
Show/Hide Answer
  1. Square meters for a large window, square centimeters for a small window
  2. Square meters
  3. Square centimeters
  4. 21.45 m2
  5. 10,342.8 mm2 = 103.428 cm2
  6. 10,489.05 mm2 = 104.8905 cm2

 

In this section, we will take what we have learned and apply the concepts to new situations.

Perspectives

  1. A 1 liter can of paint covers about 6 square meters. How many cans of paint should be purchased to paint a wall that measures 7.2 meters by 2.5 meters?
  2. A4 paper measures 210 mm by 297 mm. Calculate the area of a sheet of A4 paper in square centimeters.
  3. Girl with a Pearl Earring, Vermeer.

    Vermeer painted Girl with a Pearl Earring around 1665 on a canvas with dimensions 44.5 cm by 38.1 cm. What is the area of the painting?

  4. Kelly is tiling their bathroom floor which measures 3.4 m by 4.8 m. The tiles they have chosen are square and measure 0.4 m. They can be purchased in boxes of 12 tiles. How many boxes of tile should Kelly buy?
  5. Bobby wants to add chair rail around her bedroom. Her bedroom measures 4.26 m by 4.78 m. It has two doorway openings that each measure 80 cm wide. Chair rail is sold in lengths of 3 m. How many meters of chair-rail does she need to buy? How many pieces of chair rail does she need to buy?
  6. Rusty has a bookshelf that measures 76 cm. How many books of width 2.6 cm can be arranged vertically on the bookshelf?
  7. Abdou owns a bicycle with a set gear ratio that is set so that every full revolution of the pedals results in 2 revolutions of the tires. If the tires have a diameter of 700 mm, what is the distance traveled to the nearest meter by the bike in 30 full revolutions of the pedals?  HINT: the circumference of the tire can be found using the formula Circumference = π · Diameter. 
  8. Harry is selling his house and is having it measured so he can list the square footage. The footprint of his house is a rectangle with measurements of 22.86 meters by 15.24 meters. If his house has two levels, what square footage should he list? 
  9. Sam just bought a new couch with a total length of 218.44 cm and width of 106.68 cm. How many square meters of floor space will the couch take up? 

 

Show/Hide Answer
  1. 3 liters = 3 cans
  2. 1695.45 cm2
  3. 1735.5 cm2
  4. 9 boxes
  5. 16.48 m; 6 pieces
  6. 29 books
  7. 132 meters
  8. 697 m2
  9. 2.33 m2

 

Skills IconIn this section, we will use what we have learned so far to practice skill problems.

Skills Exercises

Convert the measurements:

  1. 456 cm to meters
  2. 78,390 mm to meters
  3. 82,000 m to km
  4. 3.5 km to meters
  5. 4.6 m to decimeters
  6. 2.3 km to hectometers
  7. 7.4 dekameters to centimeters
  8. 19,300,000 mm to km
  9. 3m2 to square centimeters
  10. 80,000 cm2 to square meters
  11. 3.2 km2 to m2
  12. 500,000 mm2 to m2
  13. 3,400,000,000 cm2 to km2
  14. 5.5 dm2 to cm2
  15. 87.4 m2 to dam2
Show/Hide Answer
  1. 4.56 m
  2. 78.39 m
  3. 82 km
  4. 3500 m
  5. 46 dm
  6. 23 hm
  7. 7400 cm
  8. 19.3 km
  9. 30,000 cm2
  10. 8 m2
  11. 3,200,000 m2
  12. 0.5 m2
  13. 0.34 km2
  14. 550 cm2
  15. 0.874 dam2

 

 

 

  1. https://usma.org/origin-of-the-metric-system ↵
definition

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Numeracy Copyright © 2023 by Utah Valley University is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.