CHAPTER 3: RELATIVE MEASUREMENT

3-4: Percentages in Life

Part of a Whole

When three quantities are related through a formula, knowing two of the three quantities always allows us to find the third. So, since Percent is Part of a Whole, knowing two of the three always leads to the third. As a formula,

\text{Percent}=\dfrac{\text{Part}}{\text{Whole}}    or    \text{Part}=\text{Percent} \times \text{Whole}.

Explore Icon The Appropriate Use of Percent

A percent of a measurement is defined as the measurement of Part  divided by the measure of Whole. For example, if Todd ate 4 out of 8 slices of pizza, then the percent of the pizza that he ate is the part that he ate (i.e., 4 slices) divided by the the whole pizza (i.e., 8 slices). So percent = \dfrac{4\;slices}{8\;slices}=\dfrac{1}{2}=50\%. When calculating a percent, it is vital to correctly identify the part and the whole. Otherwise, the percent will be meaningless.

 

Explore 1 – Identifying the Part and the Whole

  1. In a survey of 120 Americans, 11 stated they owe a medical bill and 109 stated they do not. Goku claims that the percentage of people that owe a medical bill is 11/109. Do you agree with Goku’s answer? Explain your reasoning.

Solution

Goku’s answer of 11/109 consists of the 11 Americans who owe a medical bill and the 109 Americans who do not owe a medical bill. Goku’s answer should be the 11 Americans who owe a medical bill compared to the 120 total Americans who were surveyed. So, \dfrac{11}{120} \approx 9.2\% of Americans owe a medical bill.

2. The student to faculty ratio at Utah Valley University averages 24:1. Ted claims that the percentage of faculty is 1/24 = 4.2%. Do you agree with Ted’s answer? Explain your reasoning.

Solution

A student to faculty ratio of 24:1 means that for every 24 students there is (on average) 1 faculty member. Ted’s answer of 4.2% consists of \dfrac{\text{Number of faculty}}{\text{Number of students}}=\dfrac{1}{24}. This tells us that the student population is 24 times as big as the faculty population (or the faculty population is 1/24th of the student population).

However, if there are 24 students to 1 faculty member, there are 25 people total in this ratio. So the percentage of faculty out of all students and faculty is actually 1 out of 25 people = 4%.

 

Explore 2 – Determining percentages

1. Sophia wants to know the percentage of time she has to relax with her family on a typical work day. Sophia spends 7 hours sleeping, 1 hour getting ready for work in the morning, 8 hours at the office, 1-hour total commute time to the office and back, and 2 hours cooking and cleaning up. What is the percent of time Sophia has to solely spend and relax with her family? (Round to the nearest whole percent).

Solution

The total time Sophia spends “working” = 7 hr + 1 hr + 8 hr + 1 hr + 2 hr = 19 hr. Since there are 24 hours in the day, Sophia has 24 hr – 19 hr = 5 hr to spend relaxing with her family. So, the percent of time Sophia has for relaxing is \dfrac{5\;hr}{24\;hr}=20.8\overline{3}\%\approx21\%

2. Jamal found a great deal when he paid $198.40 per night for his hotel room in Park City. The tax paid on the room was $23.40. What is the room tax percentage in Park City?

Solution

In this case, the whole is the room rate and the part is the tax. So room tax percentage = \dfrac{tax}{room\;rate}=\dfrac{\$23.40}{\$198.40}=0.1179...\approx11.8\%

3. Kevin shops in a store that holds sales of 40% to 70% off. Kevin sees a desk lamp that has a regular price of $25 and a sale price of $15. What is the percent of discount on the desk lamp?

Solution

The discount on the lamp is $25 – $15 = $10. The discount is taken off the regular price, so the percent of discount is taken against the regular price:

Percent of discount = \dfrac{\text{Discount}}{\text{Regular price}}=\dfrac{\$10}{\$25}=0.4= 40\%

The lamp was discounted 40%.

 

Examples

  1. In a survey 57% of Gen Z gamers feel they’re able to self-express more openly to others in a game than they do in real life. Identify the whole, the part, and the percent.[1]
  2. In a survey of young Americans aged 11 – 24, 54% said they’ve never had the simple joy of seeing a cow in real life.[2] Identify the whole, the part, and the percent.
  3. Michael buys a laptop on sale for $999.99. Its original price was $1299.99. a) Calculate the discount. b) Calculate the percentage discount off the original price.

 

Show/Hide Answer
  1. Whole = Gen Z gamers who took the survey; Part = Gen Z gamers who took the survey who feel they’re able to self-express more openly to others in a game than they do in real life; Percent = 57% = (Gen Z gamers who took the survey who feel they’re able to self-express more openly to others in a game than they do in real life)/(Gen Z gamers who took the survey)
  2. Whole = Americans aged 11 – 24 who took the survey; Part = Americans aged 11 – 24 who took the survey and said they’ve never seen a cow in real life; Percent = 54% = ratio of (Americans aged 11 – 24 who took the survey and said they’ve never seen a cow in real life)/(Americans aged 11 – 24 who took the survey)
  3. a) $1299.99 – $999.99 = $300    b) \dfrac{\$300}{\$1299.99}=23\%

 

Reflect Icon

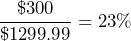
  • There are two kinds of ratio. One is part-to-part and the other one is part-to-whole. Does it make sense to turn a part-to-part ratio to a percentage? For example, a recipe has the ratio 2 to 5 of sugar to flour. Does the percentage 40% make sense by dividing 2 by 5 (i.e., 2/5=0.4=40%)? Explain your reasoning.
Show/Hide Answer

It makes sense if you claim the amount of sugar is 40% of the amount of flour. It is incorrect if you claim the amount of sugar is 40% of the recipe because the sugar is not 40% of the recipe. The recipe consists of flour + sugar + other ingredients.

 

Practice Exercises

  1. A study of the all-time scoring leaders in the NBA (as of March 2023) shows that LeBron James leads with 38,450. In comparison, Michael Jordan had 32,292 points during his career.[3] a) How many more points does LeBron James have over Michael Jordan? b) Calculate the percent of points LeBron James has more than Michael Jordan.
  2. A large department store is advertising a sale of “Up to 60% off!”. Hayley bought a dress for $47.60 that was originally priced at $68.00. a) How much did Hayley save? b) What percent off the original price did Hayley get?
  3. A patchwork linen blend teddy bear is on sale for $88. It was originally priced at $220. Calculate the percent off the sale price is from the original price.
  4. A recent grocery store bill was $235.64. This included tax of $7.97. Calculate the tax rate on this bill.
Show/Hide Answer
  1. a) 6158  b) 19%
  2. a) $20.40  b) 30%
  3. 60%
  4. 3.4%

 

Explore Icon Finding the Part and the Whole

A percentage is defined as the Part divided by the Whole, or Percent=\dfrac{Part}{Whole}.

A rearranged version of this formula is Part=Percent \times Whole, which is derived from multiplying both sides of the equation Percent=\dfrac{Part}{Whole} by Whole \left(i.e., \cancel{Whole}\times\dfrac{Part}{\cancel{Whole}}=Percent \times Whole\right). We may use this rearranged formula to find the part if given the whole and the percentage.

Another rearranged version of this formula is Whole=\dfrac{Part}{Percent}, which is derived from the formula Part=Whole \times Percent by dividing both sides of the formula by Percent. We may use the formula Whole=\dfrac{Part}{Percent} to find the whole if given the part and the percent.

 

Explore 3 – Finding the Part

1. The sales tax paid on groceries in Utah is 3%. Calculate the sales tax paid on $226.53 worth of groceries. Round to the nearest cent. (Tax percent = Tax / Price)

Solution

We are given the percent (3%) and the whole ($226.53). We need to find the part: Part=Percent \times Whole

 \begin{aligned}\text{Sales tax}&=\text{Percent}\times \text{Whole}\\\\&=3\%\times\$226.53\\\\&=0.03\times\$226.53\\\\&=\$6.80\end{aligned}

 

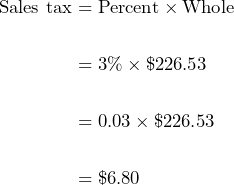
2. Marsha’s financial adviser recommended that she save 15% of her income each month. If her monthly income is $2400, how much should she place into savings?

Solution

We are given the percent (15%) and the whole ($2400). We need to find the part: Part=Percent \times Whole

\begin{aligned}\text{Savings}&=\text{Percent}\times \text{Whole}\\\\&=15\%\times\$2400\\\\&=0.15\times\$2400\\\\&=\$360\end{aligned}

 

3. The sales tax paid at a restaurant for food in Orem is 8.25%. Calculate the sales tax paid on a meal that cost $12.50.

Solution

We are given the percent (8.25%) and the whole ($12.50). We need to find the part: Part=Percent \times Whole

\text{Sales tax}&=\text{Percent}\times \text{Whole}\\\\&=8.25\%\times\$12.50\\\\&=0.0825\times\$12.50\\\\&=\$1.03

 

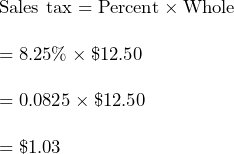
4. Three recommended percentages for leaving a tip, 18%, 20% and 25%, are shown on the payment screen as Jane pays for her dinner which cost $32.74. Calculate the recommended tip amounts for the three percentages.

Solution

We are given the percent (18%, 20%, 25%) and the whole ($32.74). We need to find the part: Part=Percent \times Whole

Tip @18% = \text{Percent}\times \text{Whole}=18\%\times\$32.74=0.18\times\$32.74=\$5.89

Tip@20% = \text{Percent}\times \text{Whole}=20\%\times\$32.74=0.2\times\$32.74=\$6.55

Tip@25% = \text{Percent}\times \text{Whole}=25\%\times\$32.74=0.25\times\$32.74=\$8.19

The recommended tip amounts are $5.89 for a 18% tip, $6.55 for a 20% tip, and $8.19 for a 25% tip.

 

Explore 4 – Finding the Whole

1. Tammy tells her mom she saved $25 after shopping in a clothing store with a 20% off promotion.

a) What was the original price of the clothes?

b) How much did Tammy spend on the clothes?

Solution

a) It is often useful to use a diagram to determine what we are given and what we need to find: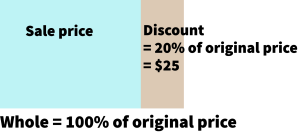       

We are given the percent (20%) and the part = discount ($25).

We need to find the whole: Whole=\dfrac{Part}{Percent}

\begin{aligned}\text{Original price}&=\dfrac{\text{Part}}{\text{Percent}}\\\\&=\dfrac{\$25}{20\%}\\\\&=\dfrac{\$25}{0.2}\\\\&=\$125\end{aligned}

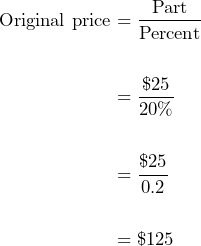
b) Since the original price was $125 and Tammy saved $25, she spent $125 – $25 = $100.

 

2. Jack’s wife complains that Jack spends too much money on entertainment every month. This month it was $250 dollars. Jack tells his wife it is just 5% of his monthly salary.  How much money does Jack earn every month?

Solution

We are given, part = $250 and percent = 5%.

We need to find the whole:   

\begin{aligned}\text{Salary}&=\dfrac{\text{Part}}{\text{Percent}}\\\\&=\dfrac{\$250}{5\%}\\\\&=\dfrac{\$250}{0.05}\\\\&=\$5000\end{aligned}

 

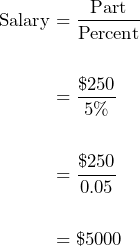
3. Alma said she just paid $20,000 in Federal taxes for last year’s income. Her Federal tax rate was 24%. How much did Alma earn last year?

Solution

We are given part = $20,000 and percent = 24%.

We need to find the whole: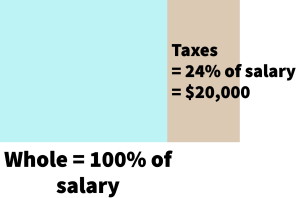

\begin{aligned}\text{Salary}&=\dfrac{\text{Part}}{\text{Percent}}\\\\&=\dfrac{\$20,000}{24\%}\\\\&=\dfrac{\$20,000}{0.24}\\\\&=\$83,333\end{aligned}

 

4. Hoa just got promoted and her new salary is $36,000. This new salary is 300% of her original salary. What was Hoa’s original salary?

Solution

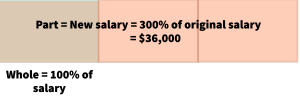We are given part = $36,000 and percent = 300%. We need to find the whole:

 \begin{aligned}\text{Original Salary} &=\dfrac{\text{Part}}{\text{Percent}}\\\\&=\dfrac{\$36,000}{300\%}\\\\&=\dfrac{\$36,000}{3}\\\\&=\$12,000\end{aligned}

 

 

Reflect Icon

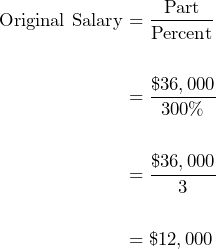
  • Is it true that the part is always smaller than the whole? Explain your reasoning.
Show/Hide Answer

It is not true. Based on the fourth question in explore 4, the part = the new salary (i.e., $36,000) is larger than the whole = the original salary (i.e., $12,000). This is because the percentage is greater than 100%. The part is 300% = three times the whole. Therefore, the part is greater than the whole.

 

Practice Exercises

  1. In a survey of 857 people who identified as lesbian, gay, bisexual, and/or transgender (LGBT), 43.7 percent reported that discrimination negatively impacted their physical well-being.[4] a) Identify the whole, the part, and the percent. b) Calculate how many LGBT people in the survey who reported that discrimination negatively impacted their physical well-being.
  2. In a study of 2210 U.S. adults, 37% get their news from social media. How many in the study get their news from social media? [5]
  3. The regular price of a jacket at a store is $299. Jane waits till it goes on sale at 30% off. How much is the sales price of the jacket?
  4. According to a national poll by University of Chicago and the West Health Institute[6], 44% of about 1300 adults didn’t seek medical care when sick or injured due to cost. How many of those adults didn’t seek medical care?
Show/Hide Answer
  1. a) Whole = 857 LGBT people who took the survey; Part = LGBT people who took the survey and reported that discrimination negatively impacted their physical well-being; Percent =  43.7% = \dfrac{ LGBT people who took the survey and reported that discrimination negatively impacted their physical well-being}{857 LGBT people who took the survey} b) Number of LGBT affected = 43.7% of 857 = 375</li> <li>818</li> <li>$209.30</li> <li>572</li> </ol> </details> </div> </div> <img class="alignnone wp-image-436" src="https://uen.pressbooks.pub/app/uploads/sites/202/2023/01/Perspective-e1674778582809-300x298.png" alt="" width="101" height="100" />In this section, we will take what we have learned and apply the concepts to new situations. <div class="textbox textbox--key-takeaways"><header class="textbox__header"> <h4 class="textbox__title"><span style="color: #000000">Perspectives</span></h4> </header> <div class="textbox__content"> <ol> <li>In the first nationwide assessment of lead-based water lines, the EPA estimated that more than 647,000 water lines in Texas are made of lead — about 7% of the state's total.[footnote]<a href="https://www.houstonpublicmedia.org/articles/news/energy-environment/2023/04/05/448334/texas-has-the-fifth-highest-percentage-of-water-pipes-made-of-lead/"><small>https://www.houstonpublicmedia.org/articles/news/energy-environment/2023/04/05/448334/texas-has-the-fifth-highest-percentage-of-water-pipes-made-of-lead/</small></a>[/footnote] Calculate the total number of water lines in Texas.</li> <li>According to a survey of 5,258 students, the main factors driving students toward a particular major were their interest in the field (57 percent), career opportunities (46 percent) and their ability to make a positive impact in that field (41 percent).[footnote]<a href="https://www.insidehighered.com/news/2023/02/13/fight-student-disengagement-real-world-projects-can-help"><small>https://www.insidehighered.com/news/2023/02/13/fight-student-disengagement-real-world-projects-can-help</small></a>[/footnote] a) How many students from the survey said their interest in the field is a main factor? b) How many students from the survey said career opportunities were a main factor? c) How many students from the survey said their ability to make a positive impact in that field was a main factor?</li> <li><img class="wp-image-2036 alignleft" src="https://uen.pressbooks.pub/app/uploads/sites/202/2023/03/statistic_id258749_most-popular-global-mobile-messaging-apps-2022-300x223.png" alt="Graph showing 2,000 million people regularly use What's App and 557 million people regularly use Snapchat." width="534" height="397" />The graphic shows the number of monthly active users in millions that use the most popular mobile messenger apps.</li> </ol> a) How many more people actively use WhatsApp than Snapchat? b) What percent of the people counted on the graph actively use WhatsApp? c) What percent of the people counted on the graph actively use Snapchat? <ol> <li value="4">The base price of a 2021 Toyota Camry is $24,970. The base price of a 2021 Kia Forte is $17,890. The price of the Kia Forte is how many percent of the price of the Toyota Camry?</li> <li>In 2020, American consumers averaged $14,353 in overall debt with $807 in credit card debt.[footnote]<a href="https://www.valuepenguin.com/average-credit-card-debt"><small>https://www.valuepenguin.com/average-credit-card-debt</small></a>[/footnote] What percent of the overall debt was the credit card debt?</li> <li>According to the 2019 U.S. census report, the population of the United States was 328.2 million, where 166.7 million were females. What was the percentage of females in the United States</li> <li>The battery of a 2019 MacBook Pro can last 10 hours. Amy's MacBook Pro shows that the battery is 58% charged. Assuming the battery will charge to 100%, about how many hours has Amy used the laptop without a charger?</li> <li>In Salt Lake City, the room tax rate at a hotel is 13.82%. a) How much tax will be paid on a room that costs $220 per night? b) What will be the total cost of staying 5 nights?</li> <li>Among the Nobel Prize winners in Chemistry and Physics from 2000-2020 in the United States, 37 of them are immigrants. They represent 37% of all US winners. How many Nobel Prize winners in these categories are not immigrants to the US?</li> <li>Only 11% of the volume of an iceberg is exposed above the surface of the ocean. A medium iceberg can range in height from 16 - 45 meters above the surface of the ocean and have a length of 61 meters.[footnote]<a href="https://rwu.pressbooks.pub/webboceanography/chapter/14-2-icebergs/"><small>https://rwu.pressbooks.pub/webboceanography/chapter/14-2-icebergs/</small></a>[/footnote] Suppose such an iceberg has a volume of 45,000 cubic meters of ice showing above the surface of the ocean. a) Calculate the volume of the whole iceberg. b) Calculate the volume of iceberg below the surface.</li> <li>The annual number of burglaries in a town fell by 40% in 2021 and then by 30% in 2022. Does this mean the number of burglaries has fallen by 70% over the two year period? Explain your reasoning.</li> </ol> <details><summary style="padding-left: 40px">Show/Hide Answer</summary> <ol> <li>9,242,857 water lines</li> <li>a) 2997 b) 2419 c) 2156</li> <li>1443 million</li> <li>71.6%</li> <li>5.6%</li> <li>51%</li> <li>4.2 hours</li> <li>a) $30.40 b) $262</li> <li>67%</li> <li>a) 409,091 cubic meters b) 364,091 cubic meters</li> <li>No. The 40% reduction is based on the base number of burglaries that happened in 2021, while the 30% drop is calculated based on the number of burglaries that happened in 2022. Since they have different bases, the percentages cannot be added. For example, if there were 1000 burglaries to start with a 40% drop would result in a drop of 0.4(1000) = 400 burglaries to 600 burglaries in 2021. Then in 2022, a 30% drop would be a drop of [latex]0.3(600)=180 burglaries to 420 burglaries in 2022. Over the two years the drop was (1000 – 420) = 580 burglaries that is a 58% fall.

 

Skills IconIn this section, we will use what we have learned so far to practice skill problems.

Skill Exercises

  1. What is 5% of 18?
  2. What is 35% of 304?
  3. What percent of 506 is 345?
  4. What percent of 9000 is 12.000?
  5. If I 35% of an amount is 42, what is the amount?
  6. If 125% of an amount is 80, what is the amount?
Show/Hide Answer
  1. 0.9
  2. 106.4
  3. 68.2%
  4. 1.33%
  5. 120
  6. 64

 

  1. https://www.businesswire.com/news/home/20220419005232/en/Razorfish-Study-Finds-52-of-Gen-Z-Gamers-Feel-More-Like-Themselves-in-the-Metaverse-than-in-Real-Life ↵
  2. https://www.travelandleisure.com/animals/survey-americans-never-seen-a-cow-in-real-life ↵
  3. https://www.statista.com/statistics/271305/all-time-nba-scoring-leaders/ ↵
  4. https://www.americanprogress.org/article/widespread-discrimination-continues-shape-lgbt-peoples-lives-subtle-significant-ways/ ↵
  5. https://www.statista.com/chart/29327/share-of-people-that-use--and-trust-different-news-sources/ ↵
  6. https://www.westhealth.org/press-release/survey2018/ ↵

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Numeracy Copyright © 2023 by Utah Valley University is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.