The United States is one of only three countries in the world (along with Liberia and Myanmar) that still use inches and feet as measurements. These units of length, along with others listed in the table below, constitute the US customary system (aka Imperial system) of weights and measures. This system is problematic for several reasons, but the most significant issue is simply that medicine, as other scientific pursuits, is an international discipline and demands a common language. That is why the metric system (known officially by its French name, Système International d’Unités, or the abbreviation SI) is the international measurement language of science.

Remembering how many inches are in a foot, how many feet are in a yard or a mile, or the relationships between seven different units of volume can be confusing if you’re from the US, and downright impossible if you’re not. The metric system has just one unit each for length, mass, volume, time, temperature, and amount of matter. You must become familiar with the base units of the metric system; they are meter, gram, liter, second, degree Celsius, and mole.

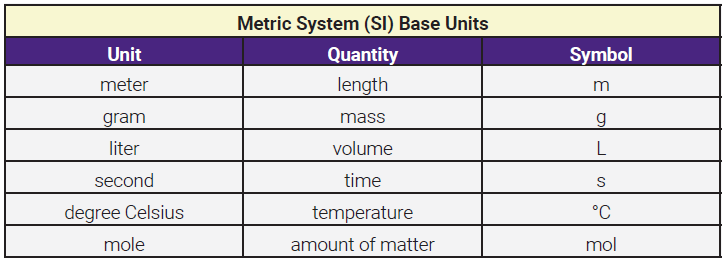
It is common to deal with very small and very large numbers in human biology and medicine. Consider mass (weight) in grams. At the atomic level, a single hydrogen (H) atom weighs about 0.0000000000000000000000017 g; a very small number. At the organism level, the average Japanese sumo wrestler weighs about 180,000 g; a rather large number. Both of these numbers are written in standard notation and, because it’s a drag to write all those zeroes, we rarely write them that way. Instead, using exponential notation (scientific notation), we write the mass of a single hydrogen atom as 1.7 x 10^-24 g and the mass of an average sumo wrestler as 1.8 x 10⁵ g.

The key to moving back and forth between standard notation and exponential notation is rather simple. If the standard notation number is smaller than 1 (begins with “zero point something,” i.e., 0.0…, 0.1…, 0.2…, …0.9…), the exponent (superscript number) will always be negative (e.g. 10^-5). If the standard notation number is 1, the exponent will be 0 (1 = 100). If the standard notation number is larger than 1, the exponent will always be positive (e.g. 10⁵). To determine the numeric value of the exponent, just count how many places you move the decimal point.

For example, let’s assume you need to write 0.0000052 in exponential notation. First, note it is smaller than 1, which means the exponent will be negative. Second, note where the decimal is and count how many places it must move to end up after the “5”…

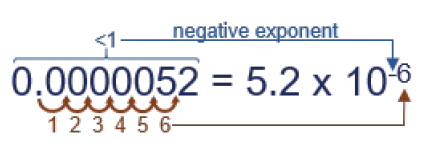
Next, maybe you need to write 63,000,000 in exponential notation. First, note it is larger than 1, so the exponent will be positive. Second, note where the decimal is (after the last zero) and count how many places it must move to end up after the “6”…

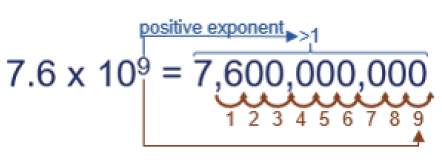
Finally, you need to write 7.6 x 10⁹ in standard notation. The exponent is positive, so the number is larger than 1. Note where the decimal is and move it 9 places…

Let’s go back to the small and large mass examples. A hydrogen atom has a mass of 1.7 x 10^-24 g and a large sumo wrestler has a mass of 1.8 x 10⁵ g. I don’t know about you, but I struggle to wrap my mind around how much is 1.7 x 10^-24 g, and 1.8 x 10⁵ g doesn’t make much sense to me either. By combining exponential notation with the metric system, it becomes even easier to express those numbers.

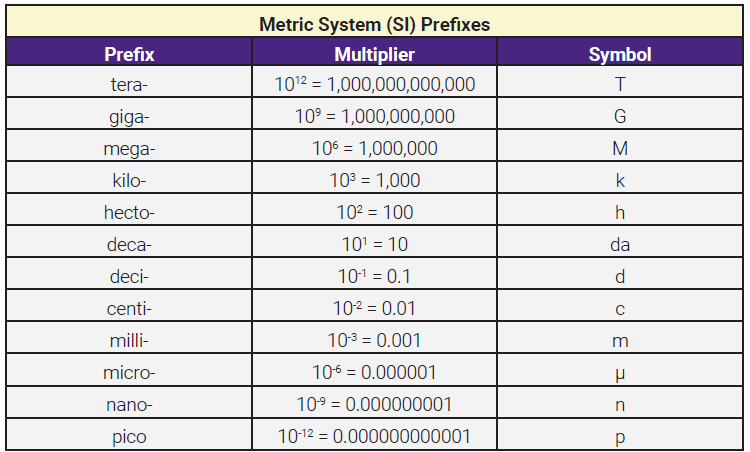
In the metric system, different powers of ten (exponents) have their own name, and those names are used as prefixes to the base units (meters, grams, liters, etc). Instead of expressing a sumo wrestler’s mass in grams (g), it makes more sense to use kilograms (kg). There are 1000 grams in one kilogram, so a sumo wrestler’s mass is about 180 kg (180,000 g = 180 kg). The prefix kilo- means 10³ (1000). Likewise, it makes more sense to use picograms (pg) for the mass of a hydrogen atom. There are 1,000,000,000,000 (1012 or 1 trillion) picograms in one gram, so a hydrogen atom has a mass of 1.7 x 10^-12 pg (1.7 x 10^-24 g = 1.7 x 10^-12 pg). The prefix pico- means 10^-12.

The prefixes are listed in this table. Notice there is not a prefix for every possible exponent (eg, none for 10⁴, 10⁵, 10⁷, 10⁸, etc). For exponents from 10^–3 through 10³ (10^-3, 10^-2, 10^-1, 10¹, 10², 10³), each exponent has a prefix. Outside that range, each third exponent has a prefix (10^–12, 10^–9, 10^–6, 10^–3; 10³, 10⁶, 10⁹, 10¹²).

At the atomic level, a hydrogen atom is about 50 pm (picometers) in diameter. At the cellular level, a red blood cell is 8 μm (micrometers) in diameter, or 160,000 (1.6 x 10⁵) times larger than a hydrogen atom. At the organism level, a typical human is 1.6 m (meters) tall, or the height of 200,000 (2 x 10⁵) red blood cells.

When we need to make a calculation that involves converting one unit to another (say, grams to picograms, or miles to kilometers), it is relatively easy to do so by applying a method called dimensional analysis or the factor-label method (or, as Mr. Fore, my high school chemistry teacher, called it, “using conversion factors”).

If we have a starting number with units, the units we want to end up with, and an appropriate conversion factor, we can apply dimensional analysis to easily make the conversion. The examples below show how to apply dimensional analysis. We’ll start with a very simple problem to demonstrate how it works, and then show you a more complex example. You will have an opportunity to practice this in Lab 1.

Example 1. Imagine you have a ruler with a label indicating it is 1.5 meters long but without any other markings, and you need to know its length in millimeters. How many millimeters are in 1.5 meters? Although you may be able to quickly solve this in your head, it’s a good example of how to use dimensional analysis.

First, and this is very important, anything divided by itself equals 1…

[latex]\color{blue} \frac{2}{2}=1\ \ \frac{5}{5}=1\ \ \frac{1000}{1000}=1\\[/latex]

…and that doesn’t apply only to numbers.

[latex]\color{blue} \frac{\text{horse}}{\text{horse}}=1\ \ \frac{\text{Anatomy&Physiology}}{\text{Anatomy&Physiology}}=1\ \ \frac{\text{meters}}{\text{meters}}=1\\[/latex]

The goal in dimensional analysis is to convert a measurement in one unit into an equivalent measurement in a different unit. We do that do that by multiplying by 1. Multiplying by 1 is always “legal” — it doesn’t change the value, but it does allow us to convert the units.

Let’s continue with Example 1 to illustrate this process. 1.5 m (meters) is equivalent to how many mm (millimeters)? Starting units = m, ending units = mm. All we need is a conversion factor.

What is the relationship between meters and millimeters? From the previous table we know that milli- means 10^-3. So…

[latex]\color{blue} 1\ \text{mm}=10^{-3}\text{m}\\[/latex] …or… [latex]\color{blue} 1\ \text{m}=10^{-3}\text{m}=1000\ \text{mm}\ \ \ \text{...so...}\ 1\ \text{m}=1000\ \text{mm}\\[/latex]

Our conversion factor, then, is 1 m = 1000 mm. Notice it contains our starting units (m) and our ending units (mm). In order to use it in our calculation, we need to write it as either…

[latex]\color{blue} \frac{1000\ \text{mm}}{1\ \text{m}}\ \text{...or...}\ \frac{1\ \text{m}}{1000\ \text{mm}}\\[/latex] …both of which are equal to 1.

Now let’s lay out the calculation. Start with what you know (1.5 m), multiply by the conversion factor, and end with the converted number in the units you want (mm).

[latex]\color{blue} 1.5\ \text{m}\ \times \text{[conversion factor]}=\text{?? mm}\\[/latex]

Insert the conversion factor (I suggest you always write this out on scratch paper).

[latex]\color{blue} 1.5\ \text{m}\ \times \frac{1000\ \text{mm}}{1\ \text{m}}=\text{??m}\\[/latex]

Notice, as you look at the whole equation, we have m (meters) as a unit above the line (1.5 m) and below the line (1 m). We have already demonstrated that meters divided by meters equals 1. In other words the two meters units “cancel each other out” (you might remember this terminology from a past math class…), leaving mm (millimeters) as the only unit in the equation.

Now, just do the math.

[latex]\color{blue} 1.5\ \text{m}\ \times \frac{1000\ \text{mm}}{1\text{m}}=\text{1500m}\\[/latex]

Remember, from above, there were two ways to write the conversion factor.

Don’t fret too much about which one to use. If you choose the correct version, your calculation will be successful; you’ll cancel out units you want to be rid of and end up with only the units you want. If you choose the wrong one, units won’t cancel out and you’ll end up with some funky units that make no sense. To illustrate, let’s try Example 1 with the other version of the conversion factor…

[latex]\color{blue} 1.5\ \text{m}\ \times\ \frac{1\ \text{m}}{1000\ \text{mm}}=0.0015\ \frac{\text{m}^{2}}{\text{mm}}\color{red} \text{?}\\[/latex]

I don’t know about you, but I have no idea what to do with units of “square meters per millimeter…”

Example 2. Let’s apply this to a common situation — child birth. When a woman goes into labor, the goal is always that she be “dilated to a 10” before a vaginal delivery. That means the woman’s cervix, which is usually either tightly closed or open only enough to allow passage of menstrual flow or sperm, has opened (dilated) much wider and now measures 10 cm (centimeters) across (diameter). If you’re not really familiar with the metric system and centimeters sounds a bit foreign, dimensional analysis can help quickly discover — 10 cm is equal to how many inches?

We will need a conversion factor that takes us from metric units of length to US customary units of length. Sometime in junior high or high school, I remember learning…

[latex]\color{blue} 1\ \text{in}=2.54\ \text{cm}\\[/latex]

To use this as a conversion factor, we write it as either…

[latex]\color{blue} \frac{1\ \text{in}}{2.54\ \text{cm}}\ \text{...or...}\ \frac{2.54\ \text{cm}}{1\ \text{in}}\\[/latex]

Next, lay out the calculation. Starting units = cm, ending units = in (inches). Plug in the conversion factor…

[latex]\color{blue} 10\ \text{cm}\ \times \frac{1\ \text{in}}{2.54\ \text{cm}}=\ ??\ \text{in}\\[/latex]

Make sure the starting units cancel out and the ending units remain and do the math…

[latex]\color{blue} 10\ \text{cm}\ \times \frac{1\ \text{in}}{2.54\ \text{cm}}=\ 3.94\ \text{in}\\[/latex]

A standard softball is 3.5 inches in diameter. Its no wonder epidurals are popular.

One final example, to illustrate how useful dimensional analysis can be.

Example 3. From my driveway in Pleasant View, Utah, to my son’s driveway in Pleasant Grove, Utah, is exactly 70 miles. Suppose, for whatever reason, I need to know that distance in kilometers. It’s not hard to find a conversion factor for miles to kilometers, but what if I can’t find one and don’t remember one? What if the only metric to US customary length conversion factor I can remember is the one I mentioned above, 1 inch = 2.54 cm, that I learned years ago? Thankfully, I can use dimensional analysis to solve the problem. (Note: We will not ask you to do anything this involved.)

Starting units = miles, ending units = kilometers, and 1 inch = 2.54 cm. What else do I know? Well…

[latex]\color{blue} 12\ \text{in}=1\ \text{ft}\\[/latex]

[latex]\color{blue} 5280\ \text{ft}=1\ \text{mile}\\[/latex]

[latex]\color{blue} 100\ \text{cm}=1\ \text{m}\\[/latex]

[latex]\color{blue} 1000\ \text{m}=1\ \text{km}\\[/latex]

You can chain together more than one conversion factor, if needed. The key is to ensure all unwanted units cancel, leaving only the units you want, then do the math…

[latex]\color{blue} 70\ \text{mi}\ \times \frac{5280\ \text{ft}}{1\ \text{mi}} \times \frac{12\ \text{in}}{1\ \text{ft}}\ \times \frac{2.54\ \text{cm}}{1\ \text{in}}\ \times \frac{1\ \text{m}}{100\ \text{cm}}\ \times \frac{1\ \text{km}}{1000\ \text{m}}=\ 113\ \text{km} \\[/latex]

Notice that miles, feet, inches, centimeters, and meters all cancel, leaving just kilometers, the unit we need. For those unfamiliar with serial multiplication, punch the above equation into your calculator as follows:

[latex]\color{blue} 70\ \times 5280\ \times 12\ \times 2.54\ \div 100\ \div 1000\ =112.65\ \approx 113 \\[/latex]